OpenSees Cloud
OpenSees AMI
The Basics of Frame Element Localization
Original Post - 25 Oct 2020 - Michael H. Scott
Show your support at Buy Me a Coffee.
Those strain softening constitutive models we like to use for concrete can lead to material nonlinearity that isolates, or localizes, in a single element or single integration point of your model. The resulting global response becomes non-objective, non-unique, or mesh-dependent.
The objective of this post is to show the localization problem then describe solutions that are available in OpenSees for force-based elements. I don’t want to get into details, I just want to show how different regularization techniques can improve localized response.
I will demonstrate localization with the reinforced concrete bridge pier shown below. Yes, it’s a well-worn example, but if I did anything more, this piece would be a journal article and not a blog post. The unconfined concrete strength is 32 MPa, and due to confinement, the core concrete strength is 39 MPa. The reinforcing steel strength is 510 MPa.

Before we get to force-based elements, let’s take a look at the response with displacement-based elements. As shown in a previous post, mesh refinement with displacement-based elements should lead to a convergent solution. However, that is not the case here. Although, as expected, the peak base shear decreases with more elements, the post-peak response is mesh dependent.
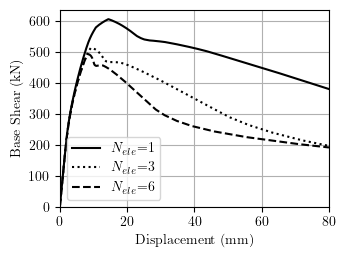
Now, turning to force-based elements, using more integration points should lead to a convergent solution. As shown below, the peak base shear is the same for all cases, but the post-peak response takes different paths as the number of Gauss-Lobatto integration points changes.
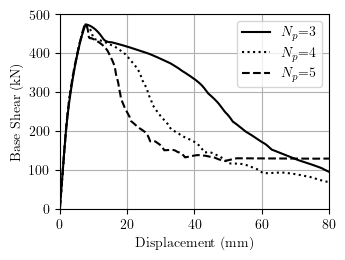
Some kind of regularization is required to obtain objective global response with force-based elements. I will show three regularization approaches.
The first approach proposed by Coleman and Spacone (2001) modifies the ultimate strain of the concrete material based on the number of integration points in order to maintain constant fracture energy release. As shown below, the results line up pretty well.

The second approach proposed by Scott and Fenves (2006) and similarly by Addessi and Ciampi (2007) modifies the integration weight at the end of the element. This allows the analyst to use an observed or empirically based plastic hinge length instead of whatever Gauss-Lobatto dictates. As shown below, the results match the first local approach.
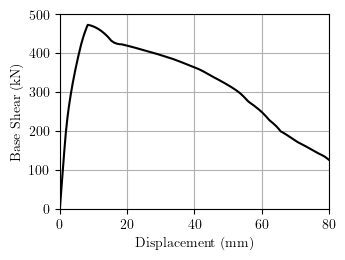
The third regularization approach is a recent addition to OpenSees. This formulation by Sideris and Salehi (2016), implemented in the GradientInelasticBeamColumn element class, uses a set of gradient-based relations to ensure continuity of section deformations over the element length. The results match the two local approaches above.
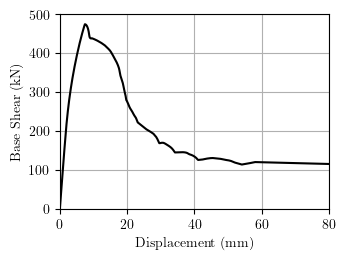
Other formulations for force-based elements were developed by Valipour and Foster (2009) and Feng et al (2015). There is also a formulation for displacement-based elements by Kenawy et al (2020). These formulations are not (yet) available in OpenSees. If you’d like to implement them, let me know!