OpenSees Cloud

OpenSees AMI

When a Deal Breaker Is Not a Deal Breaker

Original Post - 13 Dec 2020 - Michael H. Scott

Visit Structural Analysis Is Simple on Substack.

We often place too much emphasis on obtaining mathematically exact solutions for structural models. While it’s important to obtain exact solutions, e.g., for element development and comparing softwares, it’s not always necessary and definitely not always a deal breaker. The important thing is to know whether or not an exact solution is possible and the ramifications if it cannot be obtained. Here’s a case in point.

In a paper I co-authored in 2006, we examined four different approaches to plastic hinge integration in force-based frame elements: midpoint, endpoint, two-point Radau, and modified Radau. A schematic of the hinge integration approaches is shown below.

To arrive at the “best” plastic hinge integration method, we examined three criteria:

1. there is an integration point at each end of the element;
2. you can obtain the exact solution for linear-elastic prismatic beams; and
3. the integration weights at the element ends are equal to prescribed plastic hinge lengths.

Midpoint integration was thrown out for failing (1) and (2) (by a hair), endpoint integration failed (2) for giving a solution that was too flexible, and two-point Radau didn’t satisfy (3). The last method left standing was modified Radau, which as it turns out, can lead to negative integration weights in some cases.

But did we dismiss endpoint integration too swiftly because of criterion (2)? After all, it satisfied criteria (1) and (3), which are more important for localization problems. The three criteria should not be weighted equally.

Applying the four plastic hinge integration methods to the reinforced concrete bridge pier example shown in the 2006 paper and described in this post, we see the following results.

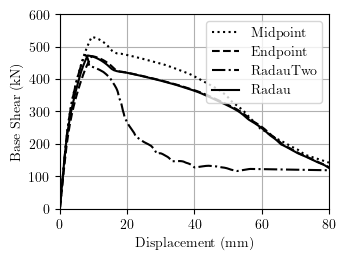
The midpoint and two-point Radau approaches remind us of their shortcomings: midpoint integration doesn’t sample at the end of the element, so the lateral load capacity is overestimated while there is rapid loss of post-peak strength because the integration weight at the end of the element is \(l_p/4\) for two-point Radau.

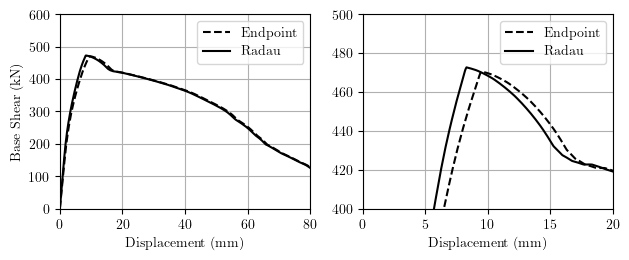
Focusing on the yield point for only endpoint and modified Radau hinge integration, we see that the yield displacement for endpoint integration is larger than that for modified Radau–by about 1.2 mm, a little more than the thickness of a dime. The peak base shear differs by about 2 kN, also due to numerical integration error.

Eigenvalue analysis after applying the gravity load, but before starting the pushover analysis, reveals the fundamental period of the bridge pier to be 0.35 sec for endpoint integration and 0.33 sec for modified Radau.

Differences of 1.2 mm, 2 kN, and 0.02 sec, and the notion of exact, are meaningless in reinforced concrete and other materials of construction. Such small differences are not limited to this specific model. Variance due to uncertain properties of construction materials is generally much more significant than errors in mathematical formulations.