OpenSees Cloud
OpenSees AMI
All About Making that ZPA
Original Post - 06 Oct 2024 - Michael H. Scott
Show your support at Buy Me a Coffee.
On a recent project, I encountered a new term: the zero period acceleration, or ZPA. Of course it’s a common term, just new to me.
The ZPA is the peak pseudo-acceleration for modes of vibration whose frequency is very high relative to the input excitation. These modes respond in-phase, or quasi-statically, with the input excitation, as if the modes have zero period.
For an earthquake excitation, the ZPA is the peak ground acceleration. That’s easy–and unfortunately, that’s not the point of this post.
The real quantity of interest is fZPA, the frequency above which the pseudo-acceleration is equal to the ZPA.
Estimating the ZPA frequency is straightforward using an earthquake response spectrum. For example, the 5% damping response spectra generated from the Tabas and Fukushmia earthquakes are shown below. The ZPA frequency is approximately 80 Hz for Tabas and 50 Hz for Fukushima.
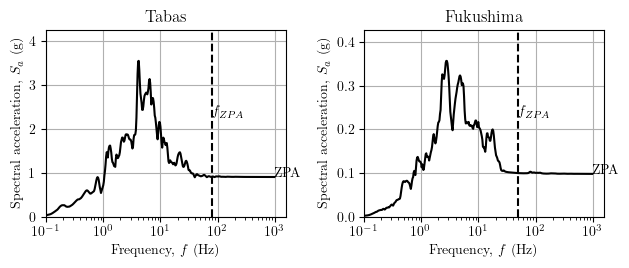
As discussed shortly, the ZPA frequency can be important if you do modal superposition or response spectrum analysis. But if you’re doing direct time integration, the ZPA frequency is not important. Just use a time step that is small enough to ensure accuracy and stability for high frequency modal response.
Further contributing to the seeming non-importance of the ZPA frequency, for realistic building models subjected to realistic ground excitations, the natural frequencies of vibration are generally much lower than the ZPA frequency and all modes respond out-of-phase with the excitation. So the ZPA frequency is a non-issue for building models, not only for direct integration, but also for modal analysis.
Consider the garden variety shear frame models that dominate earthquake engineering and structural dynamics textbooks, e.g., the 40 story shear frame from Hjelmstad (model script here). Plotted below are the model’s natural frequencies and mass participation factors along with the Tabas response spectrum (5% damping) and its ZPA frequency.
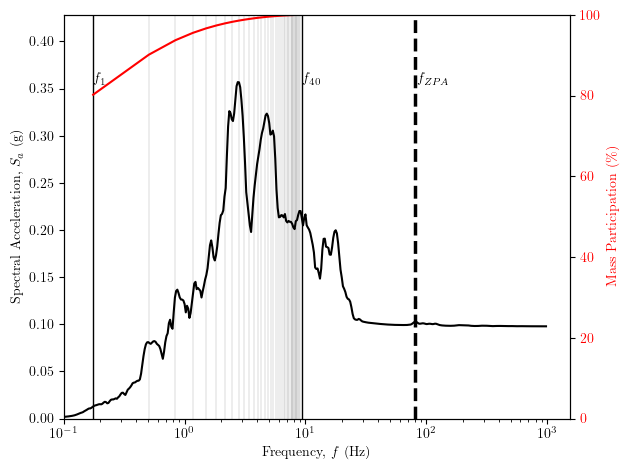
The frequencies of all 40 modes are well below the ZPA frequency and 90% mass participation is achieved with the first two modes–not uncommon for building models. Take any shear frame model from Chopra and you’ll make the same observations.
But reaching 90% mass participation can require many modes when a model has high frequencies of vibration. This was a situation I dealt with on the project where I became familiar with the ZPA.
For the model in this project, which was not about buildings, the first 30 modes account for roughly 50% of the system mass for excitation in one direction. Plus, the input excitation was of low frequency with fZPA=16 Hz, leading to dynamic response for only the first 15 modes.
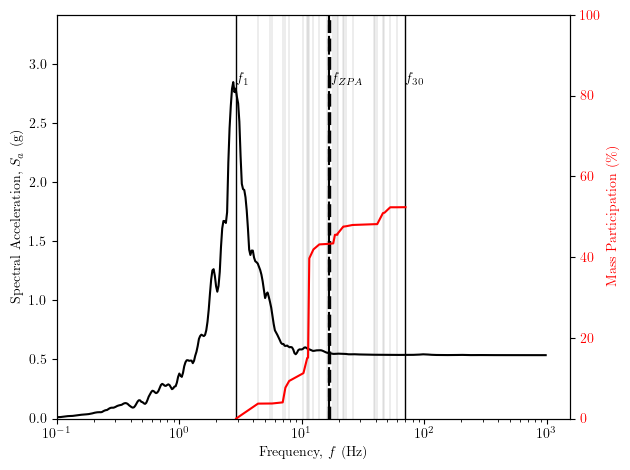
In a modal superposition or response spectrum analysis, you can lump all the modes beyond the ZPA frequency into one super mode whose response to the input excitation is quasi-static. The “static correction method” and the “mode acceleration superposition method” can handle the quasi-static response of these high frequency modes efficiently when combining modal responses. Those methods are posts for another day.
But if you, like me, learned structural dynamics through building models and have rarely used modal superposition methods since, the ZPA and its corresponding frequency could be “new” concepts to you too!